Wythoff constructions with alternations produce vertex-transitive figures that can be made equilateral, but not uniform because the alternated gaps (around the removed vertices) create cells that are not regular or semiregular. A proposed name for such figures is scaliform polytopes.[1] This category allows a subset of Johnson solids as cells, for example triangular cupola.
Each vertex configuration within a Johnson solid must exist within the vertex figure. For example, a square pyramid has two vertex configurations: 3.3.4 around the base, and 3.3.3.3 at the apex.
The symmetry order of a vertex-transitive polytope is the number of vertices times the symmetry order of the vertex figure.
The scaliforms and some related non-Wythoffian 4-polytopes are give below:
| Name | 16-cell (Snub cubic hosochoron) | Runcic snub cubic hosochoron [2][3][4] | Snub 24-cell (24-diminished 600-cell) | Runcic snub 24-cell [5][6] | 120-diminished rectified 600-cell [7][8] | Grand antiprism (20-diminished 600-cell) | bi-24-diminished 600-cell [9][10][11] |
|---|---|---|---|---|---|---|---|
| Classification | Regular | Scaliform | Semiregular | Scaliform | Scaliform | Uniform | Scaliform |
| Coxeter | s{2,4,3} | s3{2,4,3} | s{3,4,3} | s3{3,4,3} | Non-Wythoffian | ||
| Symmetry | [2+,4,3], order 48 ±1/12[T×T].2 | [3+,4,3], order 576 ±[T×T].2 | (?) order 1200 (?) ±[I×D10] | [[10,2+,10]], order 400 ? | [2[(6,2+)[2]]], order 144 ? | ||
| BSA | hex | tutcup | sadi | prissi | spidrox | gap | bidex |
| Discoverer | Schlaefli, 1850 | Richard Klitzing, 2000 | Gosset, 1900 | Richard Klitzing, 2005 | George Olshevsky, 2002? | Conway, 1965 | Andrew Weimholt, 2004 |
| Relation | 8 of 16 vertices of cubic prism, | 24 of 48 vertices of rhombicuboctahedral prism, | 96 of 120 vertices of 600-cell, | 288 of 576 vertices of runcitruncated 24-cell, | 600 of 720 vertices of rectified 600-cell, | 100 of 120 vertices of 600-cell, | 72 of 120 vertices of 600-cell, |
| Net |  |  |  |  |  |  |  |
| Images | 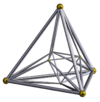  |   |   |   |   |   | |
| Vertices | 8 | 24 | 96 | 288 | 600 | 100 | 72 |
| Cells | 4+4: 6: 2: | 4+4: 6: 2: | 24: 96: 24 | 24: 96: 24: 96: | 600: 120: 120: | 100+200 20 | 48: |
| Vertex figure |  (8) 3.3.3 |  (1) 3.4.3.4 (2) 3.4.6 (1) 3.3.3 (1) 3.6.6 |  Tridiminished icosahedron (5) 3.3.3 (3) 3.3.3.3.3 |  (1) 3.4.3.4 (2) 3.4.6 (2) 3.4.4 (1) 3.6.6 (1) 3.3.3.3.3 |  Bidiminished pentagonal prism (1) 3.3.3.3 (4) 3.3.4 (2) 4.4.5 (2) 3.3.3.5 |  Bidiminished icosahedron (12) 3.3.3 (2) 3.3.3.5 |  Bitridiminished icosahedron (2) 3.3.3.5 (4) 3.5.5 |